Bài 3.10 trang 138 SBT hình học 11

Đề bài

Cho hình chóp tam giác S.ABC có \(SA = SB = SC = AB = AC = a\) và \(BC = a\sqrt 2 \). Tính góc giữa hai vectơ \(\overrightarrow {AB} \) và \(\overrightarrow {SC} \).

Lời giải chi tiết

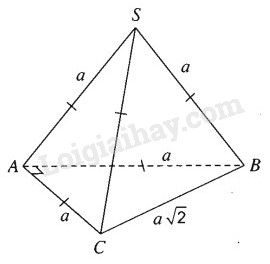
Ta tính côsin của góc giữa hai vectơ \(\displaystyle \overrightarrow {SC} \) và \(\displaystyle \overrightarrow {AB} \). Ta có

\(\displaystyle \eqalign{
& \cos \left( {\overrightarrow {SC} ,\overrightarrow {AB} } \right) = {{\overrightarrow {SC} .\overrightarrow {AB} } \over {\left| {\overrightarrow {SC} } \right|.\left| {\overrightarrow {AB} } \right|}} \cr 
& = {{\left( {\overrightarrow {SA} + \overrightarrow {AC} } \right).\overrightarrow {AB} } \over {{a^2}}} \cr & = {{\overrightarrow {SA} .\overrightarrow {AB} + \overrightarrow {AC} .\overrightarrow {AB} } \over {{a^2}}} \cr} \) 

Theo giả thiết ta suy ra hình chóp có các tam giác đều là \(\displaystyle SAB, SAC\) và các tam giác vuông là \(\displaystyle ABC\) vuông tại \(\displaystyle A\) và \(\displaystyle SBC\) vuông tại \(\displaystyle S\).

Do đó \(\displaystyle \overrightarrow {SA} .\overrightarrow {AB}  = a.a.\cos 120^\circ  =  - {{{a^2}} \over 2}\) và \(\displaystyle \overrightarrow {AC} .\overrightarrow {AB}  = 0\)

Vậy \(\displaystyle \cos \left( {\overrightarrow {SC} ,\overrightarrow {AB} } \right) = {{ - {{{a^2}} \over 2} + 0} \over {{a^2}}} =  - {1 \over 2}\)

Hay \(\displaystyle \left( {\overrightarrow {SC} ,\overrightarrow {AB} } \right) = {120^0}\)

Vậy góc giữa hai vectơ \(\displaystyle \overrightarrow {AB} \) và \(\displaystyle \overrightarrow {SC} \) bằng 120°.

 dapandethi.vn